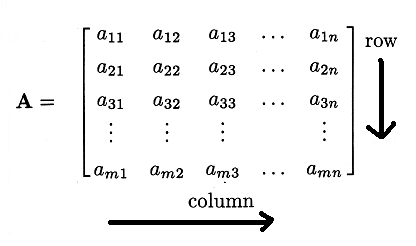
Vector norm

To measure the length of a vector is called the vector norm, in which length is measured from the origin (0,0) to the vector point on a plane. The following equation are used to measure length of a vector by 1-norm and 2-norm respectively.

1-norm Equation
The vector length is measured by the absolute sum of the vector's elements

or

2-norm Equation
The 2-norm is also called as euclidean norm and is measured by root of squared sum of the vector's elements.


or

Java code - vector norm

The following Java code finds a vector norm1 and norm2. A vector is defined in Java program by a integer array and a double array.

import java.util.Arrays;

public class Vector
 {
 
  public static double norm1(double vec[]) {  
  double nrm =0.0;
  for(int n=0;n<vec.length;n++)
   nrm += Math.abs(vec[n]);
  return nrm;
 }
 
  public static int norm1(int vec[]) {  
  int nrm =0;
  for(int n=0;n<vec.length;n++)
   nrm += Math.abs(vec[n]);
  return nrm;
 }
  
  public static double norm2 (double vec[]) {
   
   double nrm2 =0;
   for(int n=0;n<vec.length;n++)
  nrm2 += vec[n]*vec[n];  
   return Math.sqrt(nrm2);
  }
  
  public static double norm2(int vec[]) {
   int nrm2 =0;
   for(int n=0;n<vec.length;n++)
  nrm2 += vec[n]*vec[n];  
   return Math.sqrt(nrm2);
  }
 
  public static void main(String[] args) 
   {  
 System.out.println("\n Find a vector norm1 and norm2n");
 double U[]={5.0, 1.2, 2.0};      
 System.out.println( "n vector U"   );
 System.out.println( Arrays.toString(U)  );
 double nrm = Vector.norm1(U);
 System.out.println( "Vector U norm1 :" + nrm );
 double nrm2 = Vector.norm2(U);
 System.out.println( "Vector U norm2 :" + nrm2 );
  
 int V[] = {2, 5, 4};
 System.out.println( "\n vector V"   );
 System.out.println( Arrays.toString(V)  );
 int inrm = Vector.norm1(V);
 System.out.println( "vector V norm :" + inrm );           
 double inrm2 = Vector.norm2(V);
 System.out.println( "vector V norm2 :" + inrm2 );
    
  }

}

Output

Find a vector norm1 and norm2

 vector U
[5.0, 1.2, 2.0]
Vector U norm1 :8.2
Vector U norm2 :5.5172

 vector V
[2, 5, 4]
vector V norm1 :11
vector V norm2 :6.7082